Gold Nanoparticles Properties
Index of Refraction
The refractive index, denoted by "n," is a fundamental property of a material that describes how light propagates through it. It is defined as the ratio of the speed of light in a vacuum to the speed of light in the material. When light enters a medium with a different index of refraction, its speed and direction change, leading to phenomena such as reflection and refraction (Syukron & Elviyanti, 2019). The refractive index is a dimensionless quantity that quantifies the bending of light as it passes from one medium to another (Syukron & Elviyanti, 2019). It is a critical parameter in various fields such as optics, photonics, and materials science.
The refractive index is a key parameter in the study of metamaterials, which are engineered materials designed to have properties not found in nature. Metamaterials can exhibit negative refractive index, leading to unique optical properties such as negative refraction (Nellambakam & Chaitanya, 2020). The correct (positive) value of the refractive index for doubly negative metamaterials is in agreement with its definition as the length of the refraction vector (Fisanov, 2021). Furthermore, the refractive index plays a crucial role in the study of forbidden band characteristics of photonic crystals, especially those made of materials with positive and negative refractive indices (Tao, 2014).
The following table and chart shows the imaginary and real components of the index of refraction for gold as a function of wavelength. The complex refractive index m(λ) = mr(λ) + imi(λ), where λ is the free-space wavelength, mr is the real part of the refractive index, and mi is the imaginary part. The real part of a refractive index is the ratio of the free-space speed of light to the phase speed of an electromagnetic wave in the medium. The imaginary part of a refractive index is related to the absorption coefficient by 4πmi/λ. For a real refractive index, only scattering can take place. For a complex index, both scattering and absorption are possible.
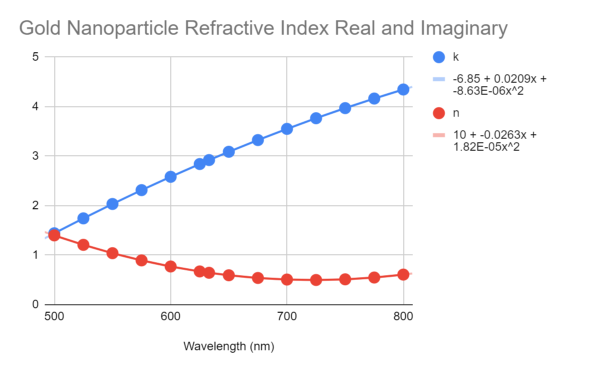
| Wavelength (nm) | k (imaginary) | n (real) |
| 500 | 1.4425 | 1.4 |
| 525 | 1.743856 | 1.208875 |
| 550 | 2.034425 | 1.0405 |
| 575 | 2.314206 | 0.894875 |
| 600 | 2.5832 | 0.772 |
| 625 | 2.841406 | 0.671875 |
| 633 | 2.921754 | 0.64464 |
| 650 | 3.088825 | 0.5945 |
| 675 | 3.325456 | 0.539875 |
| 700 | 3.5513 | 0.508 |
| 725 | 3.766356 | 0.498875 |
| 750 | 3.970625 | 0.5125 |
| 775 | 4.164106 | 0.548875 |
| 800 | 4.3468 | 0.608 |
Go here for Nanopartz Gold Nanoparticles
References:
Chiadini, F. and Lakhtakia, A. (2004). Extension of hodgkinson's model for optical characterization of columnar thin films. Microwave and Optical Technology Letters, 42(1), 72-73. https://doi.org/10.1002/mop.20212
Fisanov, V. (2021). On the sign of the refractive index for metamaterials. Russian Physics Journal, 64(8), 1560-1565. https://doi.org/10.1007/s11182-021-02490-4
Kinsler, P. (2009). Refractive index and wave vector in passive or active media. Physical Review A, 79(2). https://doi.org/10.1103/physreva.79.023839
Nellambakam, Y. and Chaitanya, K. (2020). Metamaterials and cesàro convergence. Aip Advances, 10(4). https://doi.org/10.1063/1.5144629
Syukron, A. and Elviyanti, I. (2019). Study of a silica glass sio2-na2o refractive index to fabrication of fiber optic with brewster method. Journal of Physics Theories and Applications, 3(2), 77. https://doi.org/10.20961/jphystheor-appl.v3i2.39709
Tao, L. (2014). Research on the forbidden band characteristics of the negative refraction index one-dimensional anisotropic photonic crystal.. https://doi.org/10.1109/infoseee.2014.6948120
White, I. and Fan, X. (2008). On the performance quantification of resonant refractive index sensors. Optics Express, 16(2), 1020. https://doi.org/10.1364/oe.16.001020
Zhang, Q., Zhong, L., Tang, P., Yuan, Y., Liu, S., Tian, J., … & Liu, X. (2017). Quantitative refractive index distribution of single cell by combining phase-shifting interferometry and afm imaging. Scientific Reports, 7(1). https://doi.org/10.1038/s41598-017-02797-8
Zimmennann, L., Weibel, M., Caseri, W., & Suter, U. (1993). High refractive index films of polymer nanocomposites. Journal of Materials Research, 8(7), 1742-1748. https://doi.org/10.1557/jmr.1993.1742